4th ed. Jan 2024; 3rd ed. May 2023; 2nd ed. Dec 2009; 1st ed. Sep 2004
“It is not philosophy but active experience in mathematics itself that alone can answer the question: `What is Mathematics?'” – Richard Courant & Herbert Robbins, 1941, What is Mathematics?, Oxford University Press)
“An adequate presentation of any science cannot consist of detailed information alone, however extensive. It must also provide a proper view of the essential nature of the science as a whole.” – Aleksandrov, 1956, Mathematics: Its Content, Methods, and Meaning
‘What is mathematics?’ Much ink has been spilled over this question, as can be seen from the selection of ten respected responses provided in the footnote, with seven book-length answers, and three written in the current millenium. One might well ask, is there anything new that can be said, that should be said? We’ll start by clarifying what a good answer should look like, and then explore the answer proposed.
The rest of the paper follows the structure below:
1. Criteria for a Good Definition of Mathematics
2. Definition 1: covering mathematics up to the end of the 18th century (1790s)
3. Two Perspectives
Mathematics as Dialectic (Lakatos)
Mathematics shaped by its Anthropology (Hoyrup)
4. Definition 2: covering all mathematics, including contemporary mathematics
5. The emergence of contemporary mathematical practice from 1800s onward
6. Three Facets of Mathematics
1. Mathematics as an Empirical Science
2. Mathematics as a Modeling Art
3. Mathematics as an Axiomatic Arrangement of Knowledge
7. Mathematics "from the inside": Mathematicians writing about Mathematics
8. Continue Reading
9. References
Continue reading this article…
2nd ed. June 2023; 1st ed. April 2010
The term “mathematical maturity” is sometimes used as short-hand to refer to a blend of elements that distinguish students likely to be successful in mathematics. It is a mixture of mathematical interest, curiousity, creativity, persistence, adventurousness, intuition, confidence, and useful knowledge.[1],[2],[3]
With advances in machine learning, computer science, robotics, nano-materials, and many other quantitative, fascinating subjects, students today have increasingly more choice in technical studies besides mathematics. To attract and retain mathematics students, it is important that mathematics instruction be experienced as both intellectually and culturally rewarding in addition to being technically empowering. Losing students from mathematics who are otherwise capable, engaged and hard-working is tragic when it could have been avoided.
In this article, building on observations gained over the years teaching and coaching students in mathematics, we consider how enriched mathematics instruction (inquiry-based/discovery learning, historiography, great ideas/survey approaches, and philosophical/humanist) can help (1) develop mathematical maturity in students from at-risk backgrounds and prevent their untimely departure from quantitative studies, (2) strengthen the understanding of those that are already mathematically inclined, (3) expand mathematical and scientific literacy in the wider population.
Continue reading this article…
By Assad Ebrahim, on April 15th, 2010 (13,135 views) |
Topic: Education, Maths--Philosophy
An examination of mathematical methods and the search for mathematical meaning.
This article curates a reading list (most sources available freely online) organized into a set of encounters that lie outside the standard mathematics curriculum. They are intended to enrich the reader’s understanding of mathematics and its place in scientific inquiry, increase her/his connection to the historical and philosophical questions behind the mathematics of the past and present, and gain greater satisfaction from further mathematical study. The reader should come away with a better understanding of the culture of mathematics: what mathematics is, mathematical method and meaning, and the relation of mathematics to the empirical world and to science.
We look at seven topics. These may be covered in any order, to suit your particular interests.
- What is Mathematics? (Its Nature and Characteristics)
- Reality, Truth, and the Nature of Mathematical Knowledge
- What is Proof? and the Problem of Certainty
- Some Readings in the History of Mathematics and the Evolution of Its Ideas
- The Search for Foundations in Mathematics
- Mathematics and Science
- Thoughts on Mathematical Practice and Mathematical Style
There is no core body of technical material to master in this course; the important thing is a feel for how, why, and in what context the core ideas of mathematics evolved, getting to the essence of their motivation, and understanding the fruits of these efforts. The course such as the below should appeal to all those who have an itch to scratch beneath the surface of mathematics, who find themselves asking “but why?”. It could be useful in all three tiers of education: secondary, post-secondary (undergraduate), and graduate, appropriately restructured.
- Secondary school elective: to encourage bright students in mathematics, science and technology to enter the university with a broader perspective on the mathematics they will be rapidly learning there.
- University elective course: offered as a writing-intensive seminar, intended primarily for students in the sciences and engineer: mathematics, physics, engineering.
- Graduate level course: offered in the first year of graduate school in mathematics or applied mathematics as a supplementary seminar.
Continue reading this article…
By Assad Ebrahim, on February 25th, 2010 (50,554 views) |
Topic: Mathematics, Maths--Technical
Updated! February 5, 2017
The value of zero raised to the zero power, 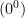 , has been discussed since the time of Euler in the 18th century (1700s). There are three reasonable choices: 1,0, or “indeterminate”. Despite consensus amongst mathematicians that the correct answer is one, computing platforms seem to have reached a variety of conclusions: Google, R, Octave, Ruby, and Microsoft Calculator choose 1; Hexelon Max and TI-36 calculator choose 0; and Maxima and Excel throw an error (indeterminate). In this article, I’ll explain why, for discrete mathematics, the correct answer cannot be anything other than 0^0=1, for reasons that go beyond consistency with the Binomial Theorem (Knuth’s argument).
Continue reading this article…
By Assad Ebrahim, on January 2nd, 2010 (111,638 views) |
Topic: Maths--Philosophy
What are the characteristics of mathematics, especially contemporary mathematics?
I’ll consider five groups of characteristics:
- Applicability and Effectiveness,
- Abstraction and Generality,
- Simplicity,
- Logical Derivation, Axiomatic Arrangement,
- Precision, Correctness, Evolution through Dialectic…
Continue reading this article…
|
Stats: 1,089,379 article views since 2010 (Aug '24 update)
Dear Readers: Welcome to the conversation! We publish long-form pieces as well as a curated collection of spotlighted articles covering a broader range of topics. Notifications for new long-form articles are through the feeds (you can join below). We love hearing from you. Feel free to leave your thoughts in comments, or use the contact information to reach us!
|
